Sheet B (Geometric Structures)
prepared by:
Joseph Malkevitch
Department of Mathematics and Computing
York College (CUNY)
Jamaica, New York 11451
email:
joeyc@cunyvm.cuny.edu
web page:
http://www.york.cuny.edu/~malk
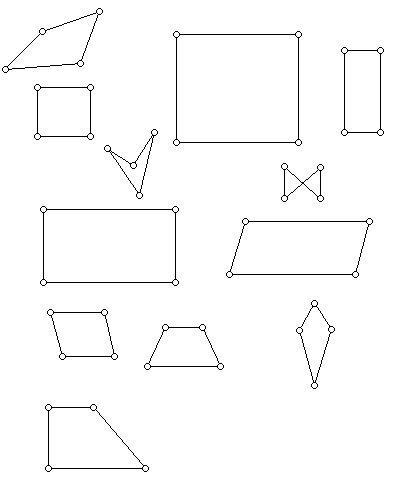
1. One can classify quadrilaterals from various perspectives:
a. Number of sides of equal length
b. Number of pairs of sides which are parallel
c. Number of right angles
d. Whether or not the "diagonals" of the quadrilateral are perpendicular.
e. Whether or not the quadrilateral is self-intersecting.
f. Whether or not the quadrilateral's "interior" is convex.
g. Is the quadrilateral equiangular?
g. Is the quadrilateral equilateral?
(Definition: A set X is convex if for any points p and q in X the line segment joining p and q is also in X.)
For each of the 4-gons below, address issues a. - f. above for that quadrilateral.

2. Can a quadrilateral have exactly 2 right angles?
3. Can a quadrilateral have exactly 3 right angles?
4. Can a self-intersecting polygon be equilateral?
5. Can a self-intersecting polygon be equiangular?